
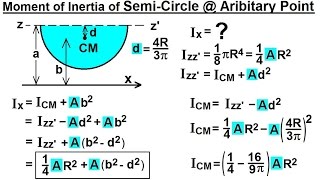
Katafygiotis is going to write and sketch with color markers directly on the board while facing you. So we is minus 30 degrees and again we can just plug into the formulas and we get that I X prime is seven pi over 32 plus squared a 3/4 8 to the fourth. The content will be primarily delivered using light board. National Soaring Museum (for content used in Chapter 9. The formula and derivation can be found in this thread. For the polar moment of inertia, which is what you would use to calculate the force for a bolt group where a moment is about the centroid of the bolt group, is Ix + Iy. Non engineering disciplines may also find the course very useful, from archaeologist who are concerned about the stability of their excavation sites to dentists interested in understanding the forces transmitted through dental bridges, to orthopedic surgeons concerned about the forces transmitted through the spine, or a hip or knee joint. In a Nut Shell: Q is the first moment of the area between the location. Circular (AC) 00-6, Aviation Weather For Pilots and Flight Operations. The moment of inertia of the bolts themselves about their individual centroids is ignored as being inconsequential. This course is suitable for learners with interest in different Engineering disciplines such as civil engineering, architecture, mechanical engineering, aerospace.
Ix prime moment of inertia of a circle how to#
You will also learn how to calculate the reaction forces as well as the internal forces experienced throughout the structure so that later you can properly design and size the foundation and the members of the structure to assure the structure’s safety and serviceability. Moment of Inertia In classical mechanics, moment of inertia, also called mass moment of inertia, rotational inertia, polar moment of inertia of mass, or the angular mass, (SI units kg m 2) is a measure of an objects resistance to changes to its rotation. the conditions under which it remains stationary or moves with a constant velocity.

In this course, you will learn the conditions under which an object or a structure subjected to time-invariant (static) forces is in equilibrium - i.e. And then if we want to find the radius of gyration we just need to take the square root of this divided by this And we get 2.Statics is the most fundamental course in Mechanics. And because it's much wider so that looks reasonable and if we had everything up we get 39.0 cubic centimeters to the fourth. Axis And this one is bigger than this one. Determine the moment of inertia and the product of inertia of a wooden T-beam section. Because it's tall this one because it's far away from the X. So, I have given some formula for the moment of inertia of different objects having a different geometrical shape. It depends on geometrical shape of the object like a circular, rectangular, triangular, thin rod, etc.
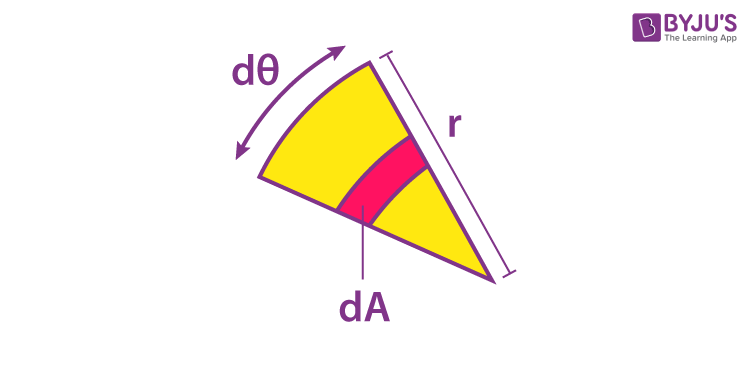
Hence the area of the circle is As rr2 7rk2 s4. The formula for the moment of inertia is different for different shapes of the object. If s denotes the distance from the fixed point O to the center of the circle, the radius of the circle, which is to vary as the square of s, must be r ks2, where k is a constant. And they seem reasonable because this has got a pretty high area moment about X. Find the volume of the frustum of the solid generated as the circle moves from s a to s b. And we just need to add all these things up. Um And then we can calculate the area moment um about X. Obtain the moment of inertia tensor of a thin uniform ring of radius R. We can calculate the Total area of this cross section and that we get 816 cm squared. principal moments of inertia about the origin and a set of principal axes. And so we have the center, we have the center the area moment about the central right? And then plus the area times this distance from the centrally to our X. And then we have the third part which is this part here. Um That's totally and so here's the values for for here. And so then we need the area of this which is B. And then we need to shift it using the parallel axis theorem from here to here.

And now for two we can take the area moment about its center of mass. So the um formula for the area moment of a rectangular shape about its central void. The moment of inertia of the shaded area is obtained by. Axis here, which is in the center of this vertical member. Compute the moments of inertia of the bounding rectangle and half-circle with respect to the x axis. So we can basically can take find the area moments for each section and then add them up to find for the total. So this part here, And then two will be this upper flank And three will be this lower flanges. So the first part, it will be the center beam here or the center section. And what we're gonna do is break it up into into three parts. So are shaded area looks something like, like what I've sketched here. Well as to determine the moment of or the second area, moment of the and the radius of gyration of the shaded area with respect to the X. JEE Main 2020 exam - Chemistry ( 9th January first shift).


 0 kommentar(er)
0 kommentar(er)
